Research
In my academic research work I use the methods of applied mathematics to investigate problems arising in physical and biological sciences, and I am particularly fascinated by Animal and Plant Biomechanics.
In recent years I have investigated models of ciliary motion, which might help shed light on new ways of detecting genetic diseases, and the nonlinear dynamics of active suspensions, which have crucial implications in cell division and growth.
I also collaborated with the Graduate School of Education on the Woodland Academy, a research project started at the University of Bristol, and focused upon developing a new curricular approach to the teaching of STEM subjects in an outdoor setting.
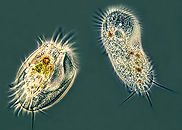
Ciliary Kinematics & Microswimmer motility



Some starting points
-
The hydrodynamics of swimming microorganisms.
Lauga, Powers, Rep. Prog. Phys. 72 (2009)
-
Synchronization, phase locking, and metachronal wave formation in ciliary chains. Niedermayer, Eckhardt, Lenz. Chaos 18 (2008)
-
Optimal kinematics of Model Cilia.
D. Cortese, Masters thesis (2012)
On small scales below millimeters, a common mechanism devised by nature to transport fluids is to exploit the time-varying deformation of flagella or cilia. Many small organisms and cells swim in a viscous environment using the active motion of these hair-like appendages which undergo periodic motion and use hydrodynamic friction to induce cellular self-propulsion. Similarly, the transport of fluid along a stationary biological channel is often achieved through the collective beating of cilia.
Modelling the way cilia move and interact with fluid flow allows us to shed light not only on biolocomotion strategies, but also on genetic diseases that can be detected by analyzing ciliary motion in tissues.
The green algae Chamydomonas is a model organism with two long cilia which is commonly experimentally observed and theoretically modelled to gain insight on how mutations affect the behaviour of cilia.


My contribution
-
Optimal kinematics of Model Cilia.
D. Cortese, Masters thesis (2012)
-
Control of helical navigation by three-dimensional
flagellar beating
D. Cortese, K.Y. Wan, Phys.Rev.Lett.. 126 (2021)
-
Distinct gaits of self-propelled quadriflagellate microswimmers
D. Cortese, K.Y. Wan, BioArXiv (2022)
-
Physics and mechanics of ciliary beating
LG Woodhams, D Cortese, PV Bayly, KY Wan.
The Chlamydomonas Sourcebook, 2023
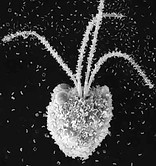
Hydrodynamics of
Active suspensions
Active materials are a new class of soft materials maintained out of equilibrium by internal energy sources. The key property that distinguishes active matter from more familiar non-equilibrium systems, such as a fluid under shear, is that the energy input that maintains the system out of equilibrium comes from the constituents, rather than the boundaries.



Probably the most promising example of an active material (with a view to applications) is the cell cytoskeleton, responsible for movement (motility) in cells.
Many other examples of such materials can be found in biological contexts: bacterial colonies, motor proteins, and the cell cytoskeleton are only some of them. Non-biological systems, for example a layer of vibrated granular rods, also display the same features.
A starting point
A really good starting point to study and explore the dynamics of active system is the following detailed review:
-
Hydrodynamics of Soft Active Matter
Marchetti et al., Reviews of Modern Physics, 85 (2013)
My contribution
-
Nonlinear spontaneous symmetry breaking in active polar films
D. Cortese, J. Eggers, T. Liverpool, Europhys J. (2016)
-
Pair creation, motion, and annihilation of topological defects in two-dimensional nematic liquid crystals
D. Cortese, J. Eggers, T. Liverpool, Phys.Rev.E (2018)
The active systems studied theoretically and experimentally so far consist of elongated self-propelled particles of two types: polar ones, with a head and a tail, and apolar (nematic) ones that are head-tail symmetric.


